Anesth Pain Med.  2018 Oct;13(4):349-362. 10.17085/apm.2018.13.4.349.

Derivation of pharmacokinetic equations

• Noh GJ

• KMID: 2435992
• DOI: http://doi.org/10.17085/apm.2018.13.4.349

Abstract

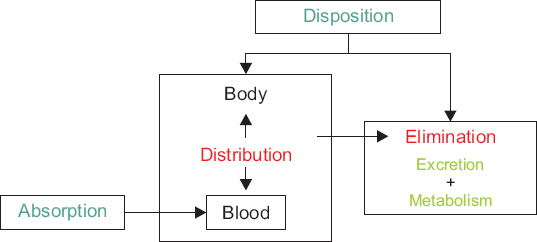
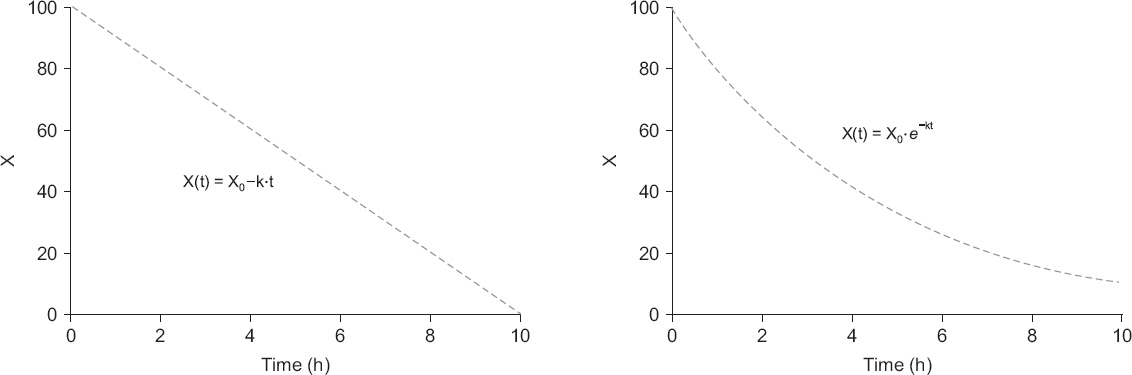
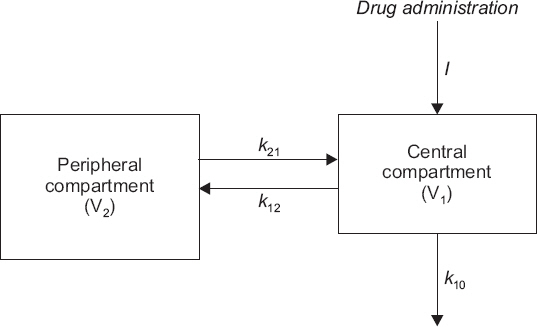
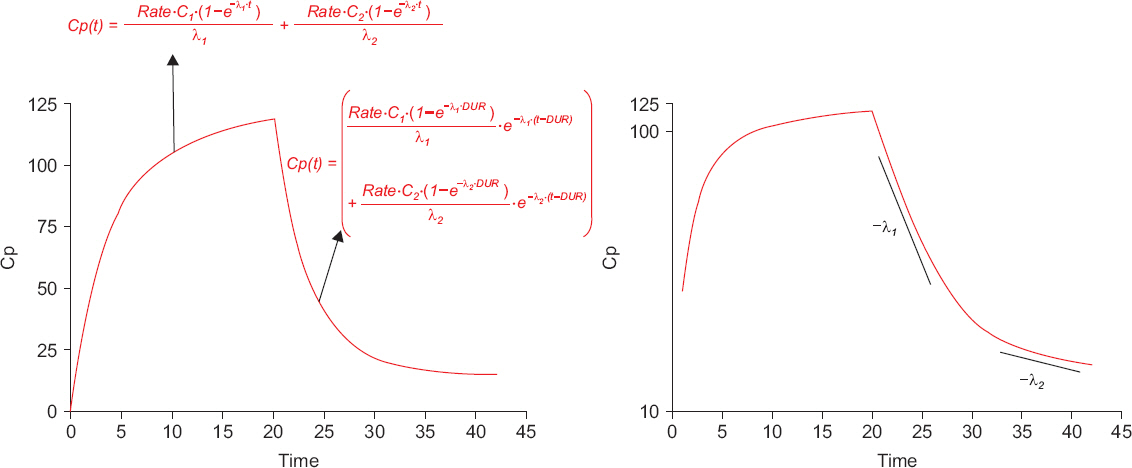
A variety of drugs are continuously or intermittently administered to patients during general or regional anesthesia. Pharmacotherapy should also receive priority compared with several treatment modalities including nerve blocks for chronic pain control. Therefore, pharmacology may be fundamental to anesthesia as well as pain medicine. Pharmacokinetic equations quantitatively evaluating drug transfer in the body are essential to understanding pharmacological principles. In mammillary compartmental models, pharmacokinetic equations are easily derived from a few simple principles. The kinetics of drug transfer between compartments is determined initially. Ordinary, linear differential equations are constructed based on the kinetics. The Laplace transforms of these differential equations are used to derive functions for the calculation of drug amounts in the central or effect compartments in the Laplace domain. The inverse Laplace transforms of these functions are used to obtain pharmacokinetic equations in time domain. In this review, a two-compartment mammillary pharmacokinetic model is used to derive pharmacokinetic equations using the aforementioned principles.

Keyword

Drug therapy; Pharmacokinetics; Theoretical models

MeSH Terms

Anesthesia
Anesthesia, Conduction
Chronic Pain
Drug Therapy
Humans
Kinetics
Models, Theoretical
Nerve Block
Pharmacokinetics
Pharmacology

Figure

• 

  Fig. 1 ADME (absorption, distribution, metabolism and excretion).

• 

  Fig. 2 Zero-order (left panel) and first-order (right panel) kinetics. X: amount of a drug. t: time in h. X0 = 100 mg, k: elimination rate constant (mgㆍh−1 for zero-order kinetics and h−1 for first-order kinetics).

• 

  Fig. 3 Two compartment mammillary pharmacokinetic model. I: input. kij: mirco-rate constant from compartment i to compartment j. Vi: volume of distribution of compartment i. Here, i = 1, 2.

• 

  Fig. 4 Time-concentration curve after an intravenous bolus of a drug following two compartment pharmacokinetic model with first-order elimination (left panel). The λ1 and λ2 are the slopes of distribution and elimination phases in the semi-log graph, respectively (right panel).

• 

  Fig. 5 Time-concentration curve after zero-order infusion of a drug following two compartment pharmacokinetic model with first-order elimination. Note that pharmacokinetic equations during and after infusion are different (left panel). Assuming that infusion duration (DUR) is 20 min, plasma concentration reaches its peak at the end of infusion (20 hours). This peak concentration is as follows. Cp(20)=Rate⋅C1⋅(1−e−λ1⋅20)λ1+Rate⋅C2⋅(1−e−λ2⋅20)λ2 Plasma concentration declines after the peak concentration. The λ1 and λ2 are the slopes of distribution and elimination phases in the semi-log graph, respectively (right panel). After the end of infusion, the followings are numerical values (DUR = 20 hours). Rate⋅C1⋅(1−e−λ1⋅DUR)λ1+Rate⋅C2⋅(1−e−λ2⋅DUR)λ2

• 

  Fig. 6 Two compartment mammillary pharmacokinetic model with an effect compartment. I: input. kij: mirco-rate constant from compartment i to compartment j. Vi: volume of distribution of compartment i. Here, i = 1, 2, 3.

Reference

REFERENCE

1. Benet LZ, Turi JS. Use of general partial fraction theorem for obtaining inverse laplace transforms in pharmacokinetic analysis. J Pharm Sci. 1971; 60:1593–4. DOI: 10.1002/jps.2600601041.