Investig Magn Reson Imaging.  2016 Mar;20(1):27-35. 10.13104/imri.2016.20.1.27.

A New Tailored Sinc Pulse and Its Use for Multiband Pulse Design

• Park J
• Park JY

• KMID: 2161367
• DOI: http://doi.org/10.13104/imri.2016.20.1.27

Abstract

PURPOSE
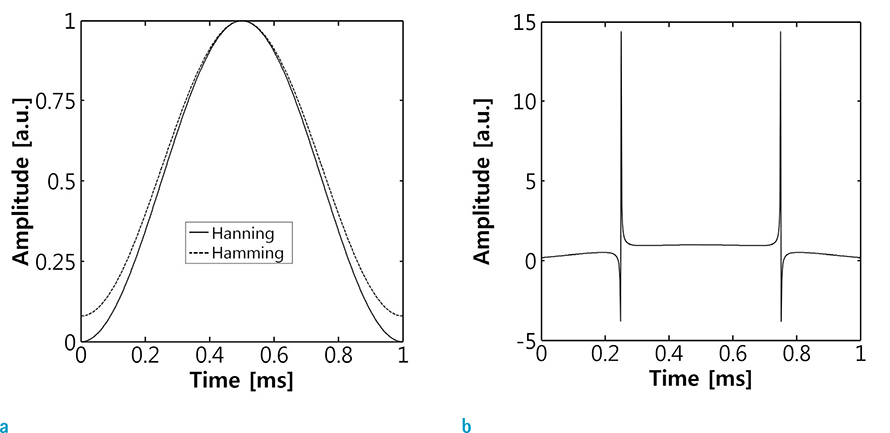
Among RF pulses, a sinc pulse is typically used for slice selection due to its frequency-selective feature. When a sinc pulse is implemented in practice, it needs to be apodized to avoid truncation artifacts at the expense of broadening the transition region of the excited-band profile. Here a sinc pulse tailored by a new apodization function is proposed that produces a sharper transition region with well suppression of truncation artifacts in comparison with conventional tailored sinc pulses. A multiband pulse designed using this newly apodized sinc pulse is also suggested inheriting the better performance of the newly apodized sinc pulse.
MATERIALS AND METHODS
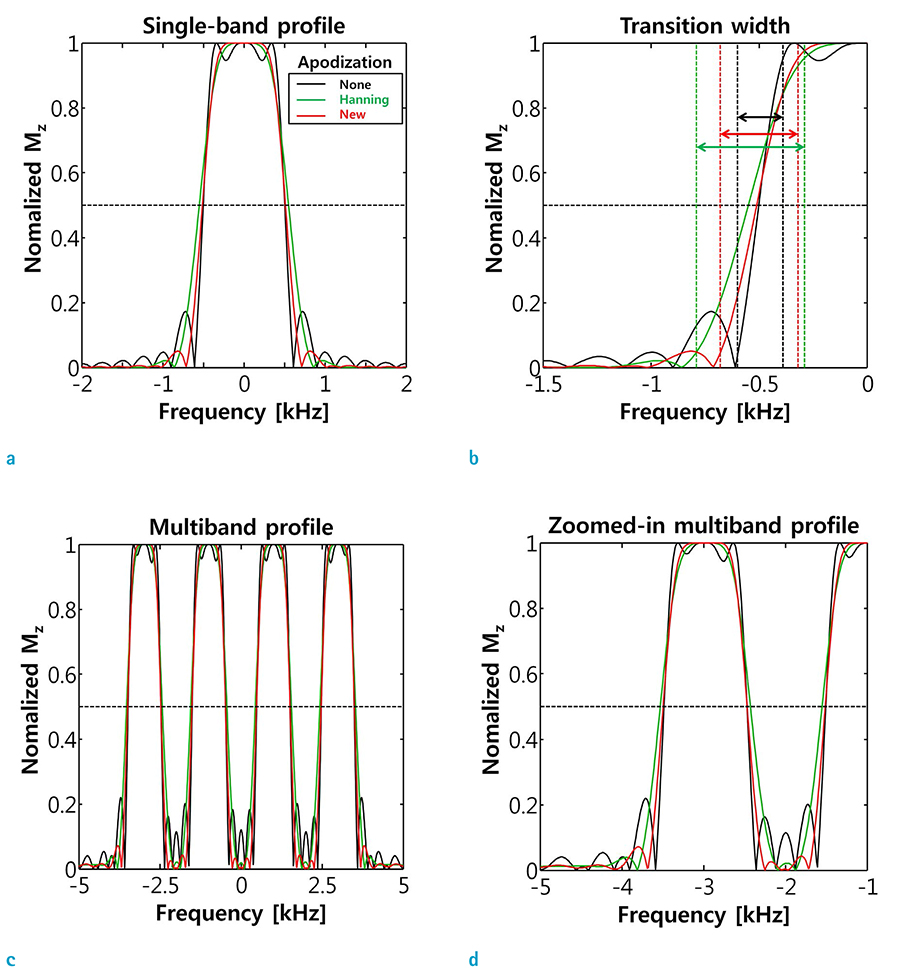
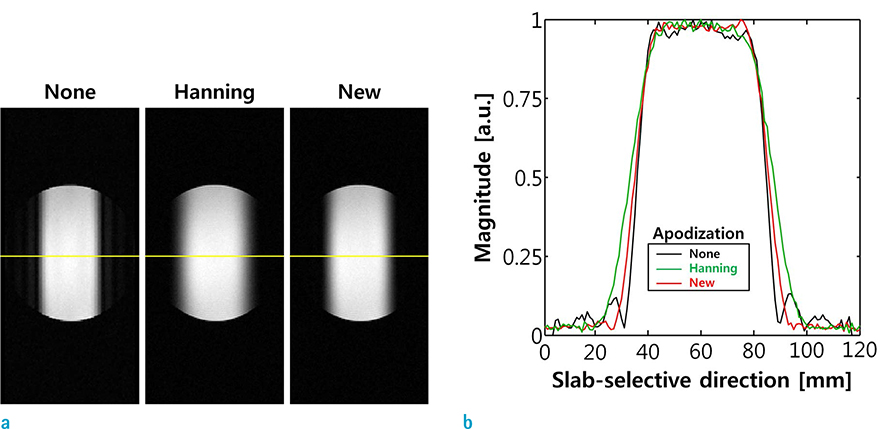
A new apodization function is introduced to taper a sinc pulse, playing a role to slightly shift the first zero-crossing of a tailored sinc pulse from the peak of the main lobe and thereby producing a narrower bandwidth as well as a sharper pass-band in the excitation profile. The newly apodized sinc pulse was also utilized to design a multiband pulse which inherits the performance of its constituent. Performances of the proposed sinc pulse and the multiband pulse generated with it were demonstrated by Bloch simulation and phantom imaging.
RESULTS
In both simulations and experiments, the newly apodized sinc pulse yielded a narrower bandwidth and a sharper transition of the pass-band profile with a desirable degree of side-lobe suppression than the commonly used Hanning-windowed sinc pulse. The multiband pulse designed using the newly apodized sinc pulse also showed the better performance in multi-slice excitation than the one designed with the Hanning-windowed sinc pulse.
CONCLUSION
The new tailored sinc pulse proposed here provides a better performance in slice (or slab) selection than conventional tailored sinc pulses. Thanks to the availability of analytical expression, it can also be utilized for multiband pulse design with great flexibility and readiness in implementation, transferring its better performance.

Keyword

Sinc pulse; Apodization function; Tailored sinc pulse; Multiband pulse; Multi-slice imaging

MeSH Terms

Artifacts
Pliability

Figure

• 

  Fig. 1 Apodization functions used for tailoring a three-lobe sinc pulse to reduce truncation artifacts. (a) Hanning- (solid line) and Hamming-apodization (dashed line) functions. (b) The new apodization function which has quite a different waveform from conventional bell-shaped apodization functions.

• 

  Fig. 2 (a) Illustration of how the new apodization function is applied to a three-lobe sinc pulse. The positions of the two sharp bipolar peaks of the new apodization function correspond to the two first zero-crossings of the original sinc pulse, shifting the first zero-crossing slightly outward from the original positions (gray arrows). (b) Comparison of sinc pulses with no apodization (black), Hanning apodization (green), and new apodization (red). While the Hanning-windowed and unapodized sinc pulses coincide in the position of the first zero-crossings, the newly apodized sinc pulse has slightly shifted first zero-crossings as expected from (a). For better demonstration, the region (0.2 ≤ t ≤ 0.3 ms) around the first-zero crossing in left side was zoomed-in at the bottom in (a) and (b).

• 

  Fig. 3 Bloch simulation results acquired using (a, b) tailored sinc pulses and (c, d) four-band multiband pulses for spin excitation. (a, b) The newly apodized sinc pulse yielded sharper transition region and narrower bandwidth at the expense of slightly larger side lobes than the Hanning-windowed sinc pulse. The amount of unwanted signals existing outside the bandwidth was the least with the newly apodized sinc pulse (Table 1). (c, d) Inheriting the characteristics of the single-band sinc pulse in each band, the four-band multiband pulse designed with the newly apodized sinc pulse showed better performance than the one based on the Hanning-windowed sinc pulse.

• 

  Fig. 4 (a) Images of a sphere water phantom acquired using three single-band sinc pulses with no apodization, Hanning apodization, and new apodization. (b) 1D magnitude profiles extracted along the horizontal yellow lines in (a). In agreement with the simulation results, the new tailored sinc pulse showed better performance with well suppression of side-lobe artifacts than the Hanning-windowed sinc pulse in terms of transition sharpness.

• 

  Fig. 5 (a) Phantom images acquired using three four-band multiband pulses designed with three sinc pulses with no apodization, Hanning apodization, and new apodization, respectively. (b) 1D magnitude profiles extracted along the horizontal yellow lines in (a). As expected from the number of bands, four selective regions were successfully excited. As was in the Bloch simulation, the multiband pulse generated by the newly apodized sinc pulse gave a better performance than the one designed using the Hanning-windowed sinc pulse. These results also demonstrate that the performance of a single-band pulse is well transferred to the multiband pulse based on that single-band pulse.

Reference

1. Pauly J, Nishimura D, Macovski A. A k-space analysis of small-tip-angle excitation. 1989. J Magn Reson. 2011; 213:544–557.

2. MacFall JR, Charles HC, Prost R. Truncated sinc slice excitation for 31P spectroscopic imaging. Magn Reson Imaging. 1990; 8:619–624.

3. Bernstein S, King KF, Zhou XJ. Handbook of MRI pulse sequences. Burlington, MA: Elsevier;2004.

4. Moeller S, Yacoub E, Olman CA, et al. Multiband multi-slice GE-EPI at 7 tesla, with 16-fold acceleration using partial parallel imaging with application to high spatial and temporal whole-brain fMRI. Magn Reson Med. 2010; 63:1144–1153.

5. Feinberg DA, Setsompop K. Ultra-fast MRI of the human brain with simultaneous multi-slice imaging. J Magn Reson. 2013; 229:90–100.

6. Wu X, Schmitter S, Auerbach EJ, Moeller S, Ugurbil K, Van de Moortele PF. Simultaneous multislice multiband parallel radiofrequency excitation with independent slice-specific transmit B1 homogenization. Magn Reson Med. 2013; 70:630–638.

7. Pauly J, Le Roux P, Nishimura D, Macovski A. Parameter relations for the Shinnar-Le Roux selective excitation pulse design algorithm [NMR imaging]. IEEE Trans Med Imaging. 1991; 10:53–65.

8. Goelman G, Leigh JS. Multiband adiabatic inversion pulses. J Magn Reson A. 1993; 101:136–146.

9. Goelman G. Two methods for peak RF power minimization of multiple inversion-band pulses. Magn Reson Med. 1997; 37:658–665.

10. Wong E. Optimized phase schedules for minimizing peak RF power in simultaneous multi-slice RF excitation pulses. In : Proceedings of the 20th Annual Meeting of ISMRM; Melbourne, Australia. 2012.

11. Park JY, DelaBarre L, Garwood M. Improved gradient-echo 3D magnetic resonance imaging using pseudo-echoes created by frequency-swept pulses. Magn Reson Med. 2006; 55:848–857.

12. Park JY, Garwood M. Spin-echo MRI using pi/2 and pi hyperbolic secant pulses. Magn Reson Med. 2009; 61:175–187.