Korean J Orthod.  2015 Jul;45(4):198-208. 10.4041/kjod.2015.45.4.198.

Effect of activation and preactivation on the mechanical behavior and neutral position of stainless steel and beta-titanium T-loops

• de Castro
• Moreira R
• Braga AC
• Ferreira A
• Pollmann M

• KMID: 2400578
• DOI: http://doi.org/10.4041/kjod.2015.45.4.198

Abstract

OBJECTIVE
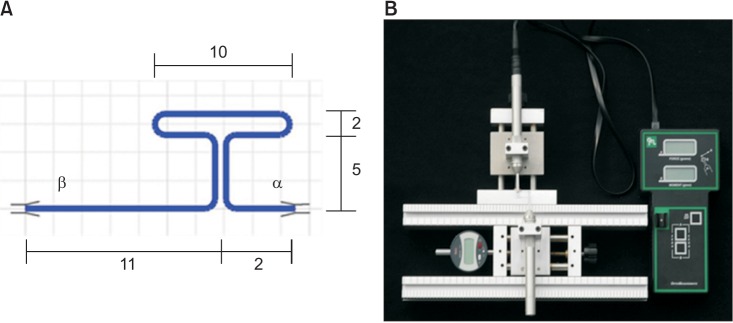
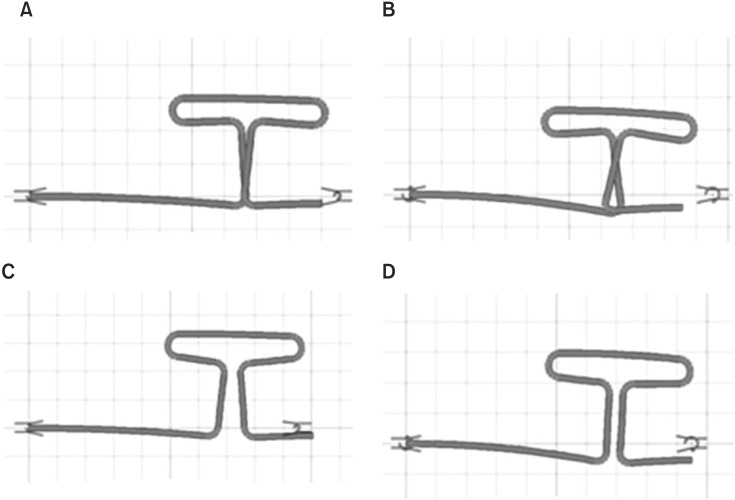
To quantify, for each activation, the effect of preactivations of differing distribution and intensity on the neutral position of T-loops (7-mm height), specifically the horizontal force, moment to force (M/F) ratio, and load to deflection ratio.
METHODS
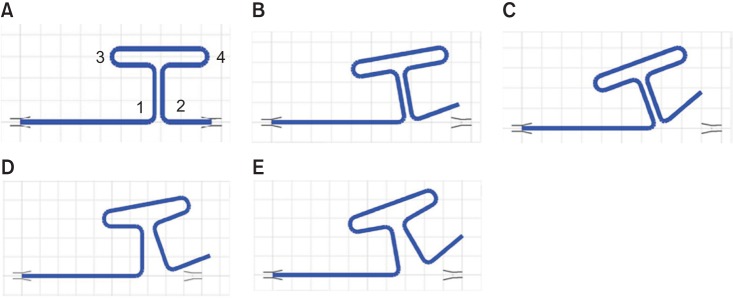
A total 100 loops measuring 0.017 x 0.025 inches in cross-section were divided into two groups (n = 50 each) according to composition, either stainless steel or beta-titanium. The two groups were further divided into five subgroups, 10 loops each, corresponding to the five preactivations tested: preactivations with occlusal distribution (0degrees, 20degrees, and 40degrees), gingival distribution (20degrees), and occlusal-gingival distribution (40degrees). The loops were subjected to a total activation of 6-mm with 0.5-mm iterations. Statistical analysis was performed using comprised ANOVA and Bonferoni multiple comparison tests, with a significance level of 5%.
RESULTS
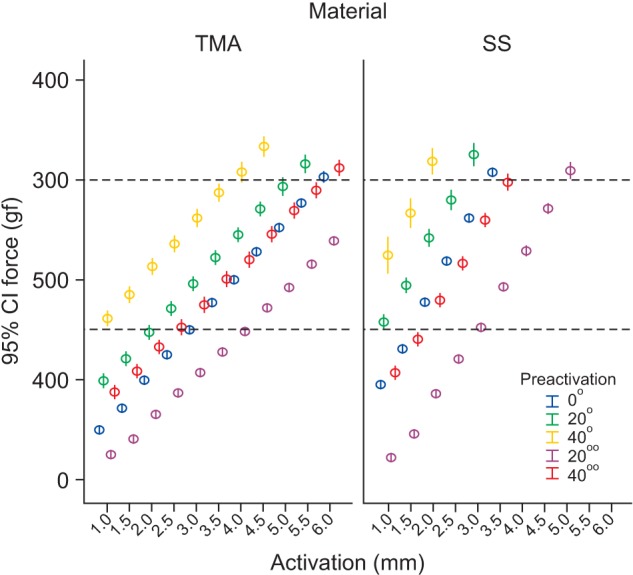
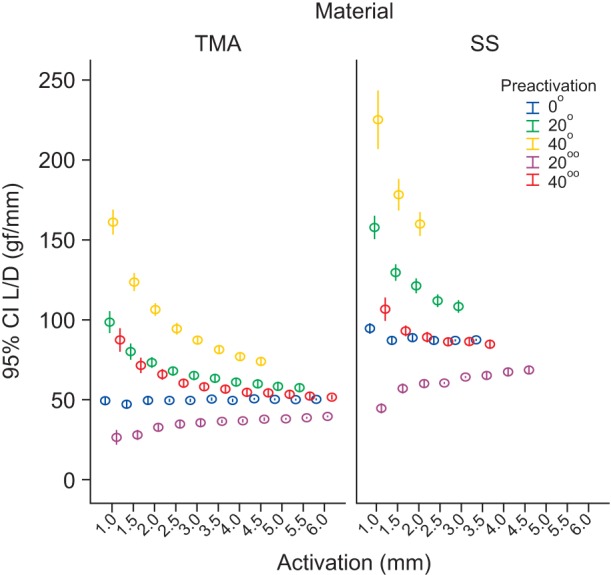
The location and intensity of preactivation influenced the force intensity. For the M/F ratio, the highest value achieved without preactivation was lower than the height of the loop. Without preactivation, the M/F ratio increased with activation, while the opposite effect was observed with preactivation. The increase in the M/F ratio was greater when the preactivation distribution was partially or fully gingival.
CONCLUSIONS
Depending on the preactivation distribution, displacement of uprights is higher or lower than the activation, which is a factor to consider in clinical practice.

Keyword

Neutral position; Preactivation; T-loop

MeSH Terms

Analysis of Variance
Stainless Steel*
Stainless Steel

Figure

• 

  Figure 1 A, Schematic representation of the T-loop and respective dimensions (mm). The front end of the loop is indicated by alpha (α), while beta (β) indicates the rear end of the loop. B, Test board: The OrthoMeasure Moment/Force/digital comparator (Orthomeasurments®; Division of Young Research & Development, Avon, CT, USA) was used to quantify the horizontal forces.

• 

  Figure 2 Schematic representation of the T-loop activation for each group. A, Numerical identification of the four bend locations; B, schematic representation of the T-loop with 20° preactivation (10° insertion in bends 1 and 2); C, 40° preactivation (20° insertion in bends 1 and 2); D, 20°° preactivation (10° insertion in bends 3 and 4) ; E, 40°° preactivation (10° insertion in bends 1, 2, 3, and 4).

• 

  Figure 3 Photograph of the inserted loop.

• 

  Figure 4 Mean force values and 95% confidence intervals (CI) for activation and preactivation in each material (beta-titanium [TMA] and stainless steel [SS]).

• 

  Figure 5 Mean moment to force (M/F) ratio values and 95% confidence intervals (CI) according to activation and preactivation in each material (beta-titanium [TMA] and stainless steel [SS]).

• 

  Figure 6 Mean load to deflection (L/D) ratio values and 95% CI by activation according to preactivation values for each material (beta-titanium [TMA] and stainless steel [SS]).

• 

  Figure 7 Schematic representation of the T-loop generated by the LOOP computer program (dHAL Orthodontic Software®, Athens, Greece). A, 20° activation/Apply Moment (neutral position); B, 40° activation/Apply Moment (neutral position); C, 20°° activation/Apply Moment (neutral position); D, 40°° activation/Apply Moment (neutral position).

Reference

1. Weinstein S, Haack DC. Theoretical mechanics and practical orthodontics. Angle Orthod. 1959; 29:177–181.

2. Vaden JL, Dale JG, Klontz HA. Aparato arco de canto de Tweed-Merrifield: filosofia, diagnóstico y tratamiento. In : Restrepo GAU, editor. Ortodoncia principios generales y técnicas. 3rd ed. Buenos Aires: Editorial Médica Panamericana S.A;2003. p. 625–682.

3. Burstone CJ, Koenig HA. Optimizing anterior and canine retraction. Am J Orthod. 1976; 70:1–19. PMID: 1066042.

4. Burstone CJ. The mechanics of the segmented arch techniques. Angle Orthod. 1966; 36:99–120. PMID: 5218678.

5. Smith RJ, Burstone CJ. Mechanics of tooth movement. Am J Orthod. 1984; 85:294–307. PMID: 6585147.

6. Siatkowski RE. Force system analysis of V-bend sliding mechanics. J Clin Orthod. 1994; 28:539–546. PMID: 8617838.

7. Braun S, Garcia JL. The Gable bend revisited. Am J Orthod Dentofacial Orthop. 2002; 122:523–527. PMID: 12439481.

8. Siatkowski RE. Continuous arch wire closing loop design, optimization, and verification. Part II. Am J Orthod Dentofacial Orthop. 1997; 112:487–495. PMID: 9387834.

9. Techalertpaisarn P, Versluis A. Mechanical properties of Opus closing loops, L-loops, and T-loops investigated with finite element analysis. Am J Orthod Dentofacial Orthop. 2013; 143:675–683. PMID: 23631969.

10. Chen J, Markham DL, Katona TR. Effects of T-loop geometry on its forces and moments. Angle Orthod. 2000; 70:48–51. PMID: 10730675.

11. Kuhlberg AJ, Burstone CJ. T-loop position and anchorage control. Am J Orthod Dentofacial Orthop. 1997; 112:12–18. PMID: 9228836.

12. Maia LG, de Moraes Maia ML, da Costa Monini A, Vianna AP, Gandini LG Jr. Photoelastic analysis of forces generated by T-loop springs made with stainless steel or titanium-molybdenum alloy. Am J Orthod Dentofacial Orthop. 2011; 140:e123–e128. PMID: 21889060.

13. Manhartsberger C, Morton JY, Burstone CJ. Space closure in adult patients using the segmented arch technique. Angle Orthod. 1989; 59:205–210. PMID: 2774296.

14. Marcotte MR. Mecânica em Ortodontia. In : Marcotte MA, editor. Biomecânica em ortodontia. 2nd ed. São Paulo: Livraria Santos Editora;2003. p. 1–21.

15. Shimizu RH, Sakima T, Pinto AS, Shimizu IA. Desempenho biomecânico da alça "T", construída com fio de aço inoxidável, durante o fechamento de espaços no tratamento ortodôntico. R Dental Press Ortodon Ortop Facial. 2002; 7:49–61.

16. Souza RS, Pinto AS, Shimizu RH, Sakima MT, Gandini LG. Avaliação do sistema de forças gerado pela alça T de retração pré-ativada segundo o padrão UNESP-Araraquara. R Dental Press Ortodon Ortop Facial. 2003; 8:113–122.

17. Siatkowski RE. Continuous arch wire closing loop design, optimization, and verification. Part I. Am J Orthod Dentofacial Orthop. 1997; 112:393–402. PMID: 9345151.

18. Thiesen G, Shimizu RH, do Valle CV, do Valle-Corotti KM, Pereira JR, Conti PC. Determination of the force systems produced by different configurations of tear drop orthodontic loops. Dental Press J Orthod. 2013; 18:19.e1–19.e18. PMID: 23916447.

19. Braun S, Sjursen RC Jr, Legan HL. On the management of extraction sites. Am J Orthod Dentofacial Orthop. 1997; 112:645–655. PMID: 9423697.

20. Halazonetis DJ. Understanding orthodontic loop preactivation. Am J Orthod Dentofacial Orthop. 1998; 113:237–241. PMID: 9484213.

21. Viecilli RF. Self-corrective T-loop design for differential space closure. Am J Orthod Dentofacial Orthop. 2006; 129:48–53. PMID: 16443478.

22. Rose D, Quick A, Swain M, Herbison P. Moment-to-force characteristics of preactivated nickel-titanium and titanium-molybdenum alloy symmetrical T-loops. Am J Orthod Dentofacial Orthop. 2009; 135:757–763. PMID: 19524835.

23. Caldas SG, Martins RP, Galvão MR, Vieira CI, Martins LP. Force system evaluation of symmetrical beta-titanium T-loop springs preactivated by curvature and concentrated bends. Am J Orthod Dentofacial Orthop. 2011; 140:e53–e58. PMID: 21803234.

24. Ferreira Mdo A, de Oliveira FT, Ignácio SA, Borges PC. Experimental force definition system for a new orthodontic retraction spring. Angle Orthod. 2005; 75:368–377. PMID: 15898375.

25. Burstone CJ, Koenig HA. Force systems from an ideal arch. Am J Orthod. 1974; 65:270–289. PMID: 4521361.

26. Ingram SB Jr, Gipe DP, Smith RJ. Comparative range of orthodontic wires. Am J Orthod Dentofacial Orthop. 1986; 90:296–307. PMID: 3464191.

27. Odegaard J, Meling T, Meling E. The effects of loops on the torsional stiffnesses of rectangular wires: an in vitro study. Am J Orthod Dentofacial Orthop. 1996; 109:496–505. PMID: 8638594.